- Home
- GCSEs: how to tackle the tricky topic of surds
GCSEs: how to tackle the tricky topic of surds

The concept of the “irrational number” is probably one of the trickier ideas we introduce in GCSE maths.
The idea of the circumference of a circle with a diameter of 1 having an immeasurable length is strange enough when you think about it. But the idea of a square with an area as simple as 2 having a length that cannot be measured is so far removed from our experience as to be seemingly absurd.
Of course, we rarely make this so explicit to our pupils, but that is actually what the reality of the situation is.
Quick read: How to build a maths curriculum
Quick listen: How to give pupils a deeper understanding of maths
Want more articles like this? Join our Tes Teaching and Learning Facebook group
The very abstract nature of irrational numbers makes it difficult to model many calculations with them in anything but a symbolic way.
For example, even explaining why something as simple as √2 × √3 = √6 is true is virtually impossible to explain without resorting to a purely symbolic exercise.
Surds made simple
This can make it hard for pupils to make sense of what is happening with lots of surd (the term being a shortened version of “absurd”) calculations, making it hard for teachers to effectively illustrate in anything approaching a concrete fashion.
There are, however, some aspects of surd calculations that can be nicely illustrated. Pythagoras’ Theorem provides a lovely way to show the progression of surd values, from √2 to √3 and so on - see the Spiral of Theodorus.
However, one method that I particularly like is to show the simplification of surds using area and squares.
Consider a square with an area of 18 units squared. The length of this square must be √18.
The square can be split into nine smaller squares, each with an area of 2.
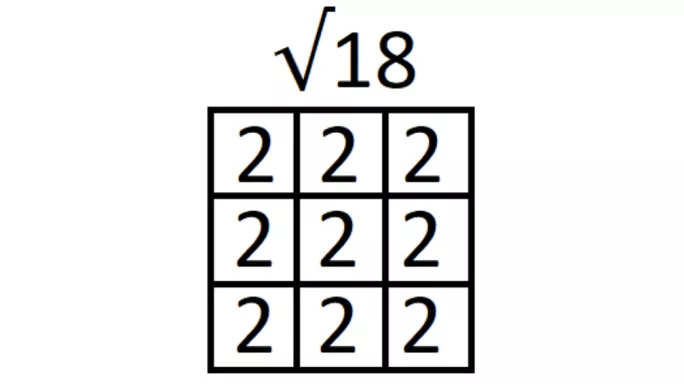
This means that each of the littler squares has a length of √2:
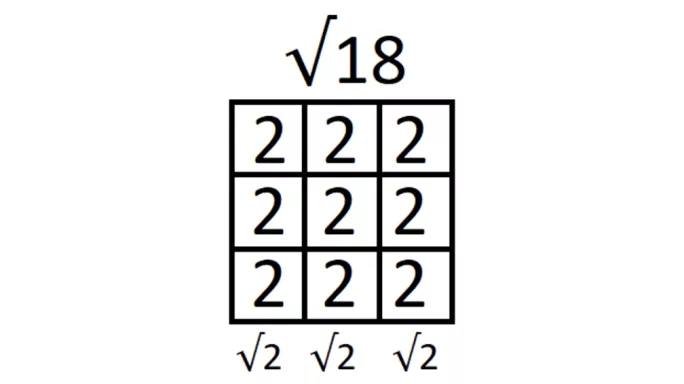
So, the result is that √18 = 3√2.
The reason I love this way of introducing the simplification of surds is because we can get a clear idea of: (a) what the decomposition of √18 into √(9 × 2) = √9 × √2 actually looks like; but also (b) we can see why not all surds simplify.
For example, if we now look at √15:
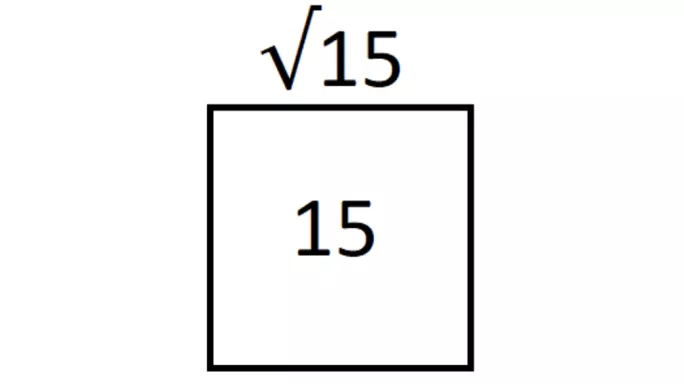
There is no way we can split this square into smaller squares, which means there is no way we can write the √15 using smaller surds.
We can split the square into rectangles, say for example into three rectangles of 5:
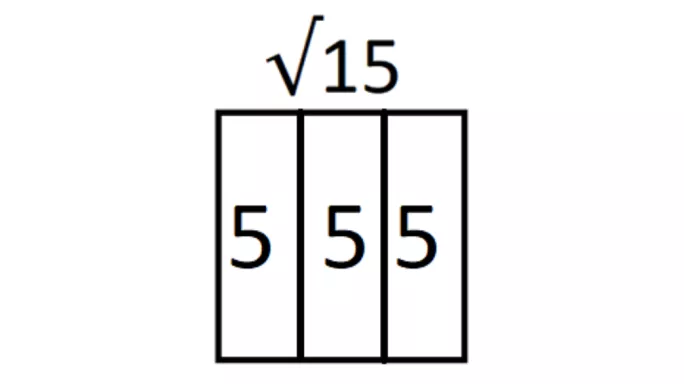
However, this doesn’t allow us to see √15 in any simpler way. It is the fact that the area of 18 can be broken into smaller squares that allowed us to find smaller surds that repeat to give the equivalent larger surd.
The same issue even occurs in larger squares, such as a square with an area of 30:
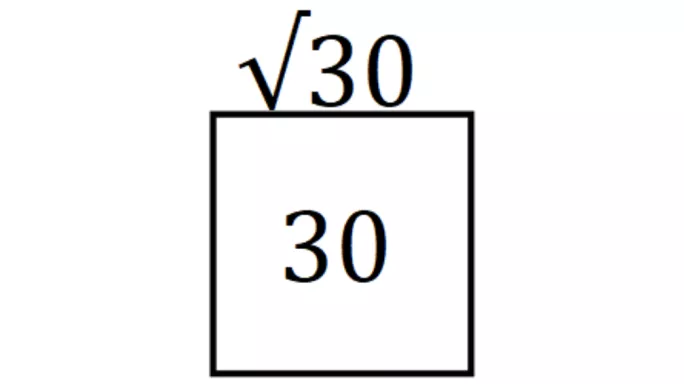
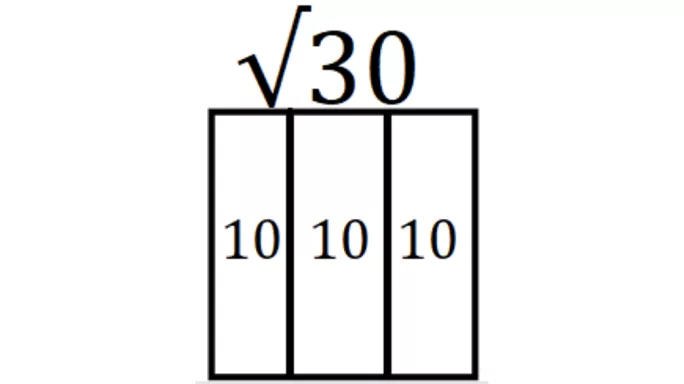
A square with an area of 30 can be split into smaller rectangles in multiple ways, for example we can split it into three rectangles of 10:
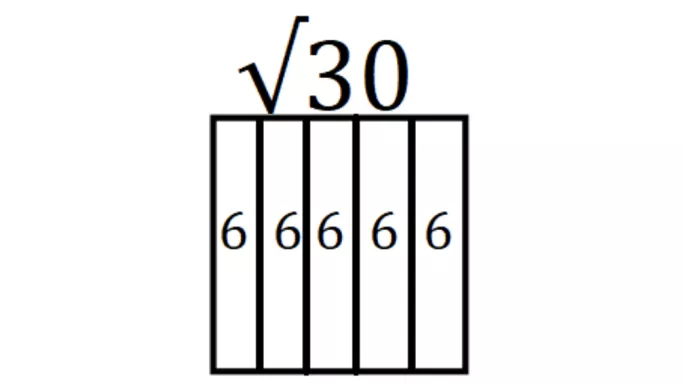
Five rectangles of six:
Or even six rectangles of five, by splitting the square into two rows of three:
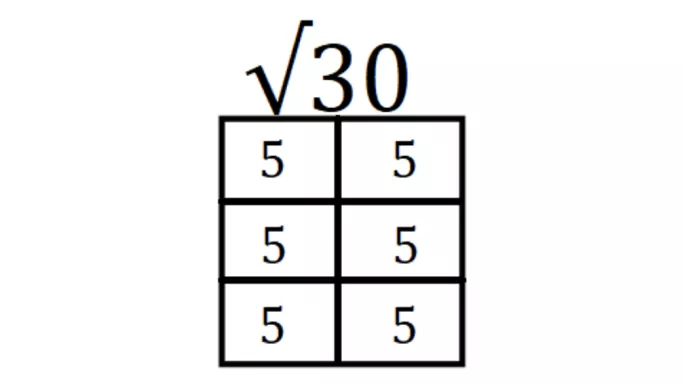
The problem is that an area of 30 doesn’t split into smaller square areas, at least not with whole values.
This makes it clear that the only time a surd will simplify is if the value inside the square root has a square factor.
We can even see why certain surds will simplify in more than one way.
For example, if we take a square with an area of 32:
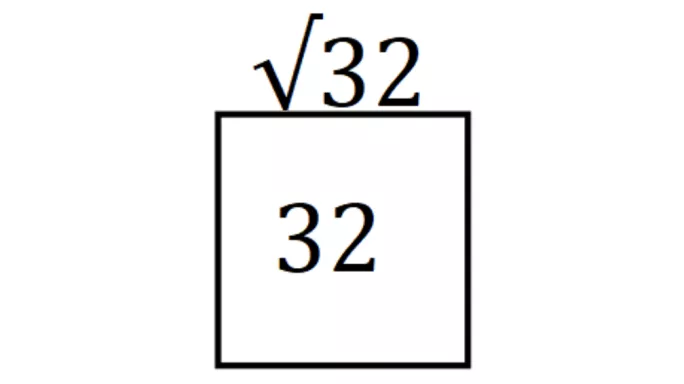
This can be broken into four squares, each with an area of eight.
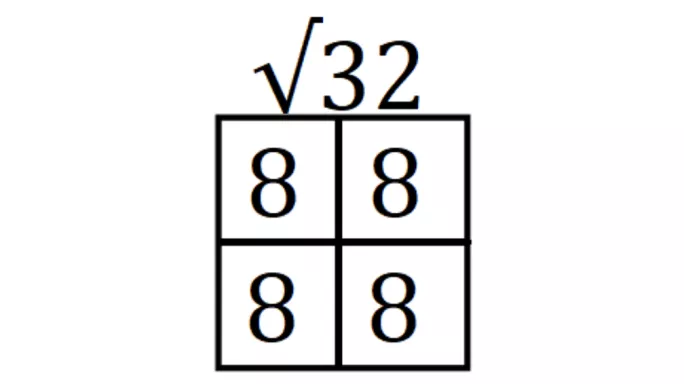
This gives √32 = 2√8.
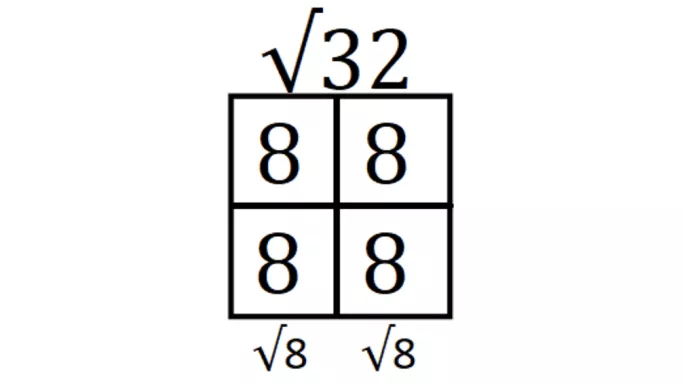
But then each of the squares with area eight can be split further into four squares with area 2:
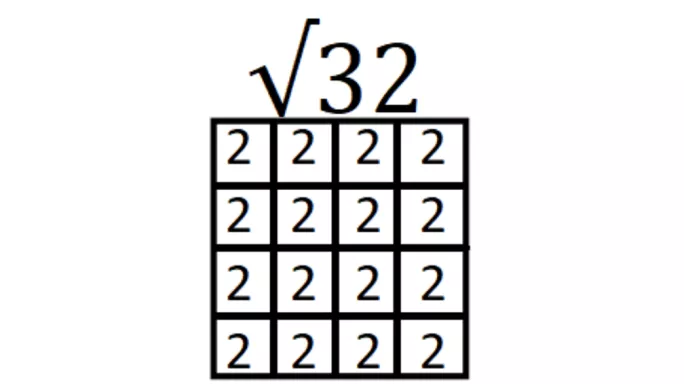
This, of course, is the same as if we have broken the original area of 32 into 16 smaller squares, each with an area of 2, and therefore a length of √2:
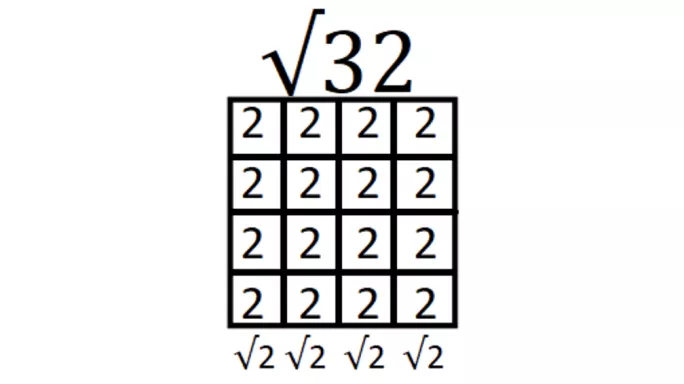
This shows that √32 can also be simplified to give 4√2.
Eventually, we would want pupils to do away with the pictures, and recognise that √32 = √(16 × 2) = 4√2.
What these images do is allow pupils to see that this is necessary, to make sense of why this is a central property of surds that simplify, and therefore to understand why the process works as it does.
Peter Mattock is head of maths at an 11-16 school in Leicestershire and author of Visible Maths
Keep reading for just £1 per month
You've reached your limit of free articles this month. Subscribe for £1 per month for three months and get:
- Unlimited access to all Tes magazine content
- Exclusive subscriber-only stories
- Award-winning email newsletters