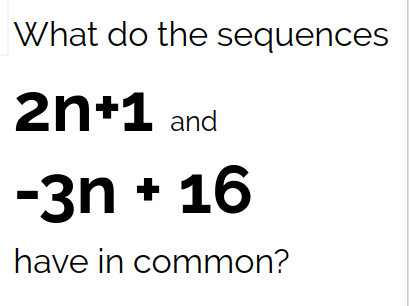73Uploads
309k+Views
248k+Downloads
Mathematics

Sequences
Exploring sequences through a series of big problems that encourage students to find multiple methods. There are additional questions to promote deeper thought and understanding around a new method or technique. We begin at finding the nth term and conclude with ‘is a term part of a sequence?’.
I’ve removed resources used to practise and consolidate methods due to restictions but all content is freely available from corbettmaths.com and variationtheory.com

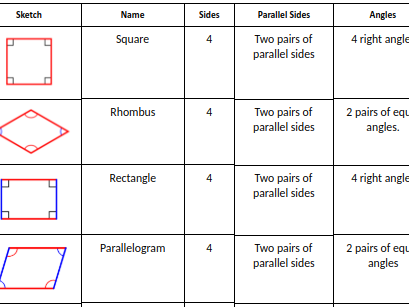
Classifying Quadrilaterals
I wasn’t quite happy with what was out there so created these 3 files. One blank, one complete(potentially a knowledge organiser for quadrilaterals) and a third for a quick quiz.
Bonus resource on Always, Sometimes, Never related to quadrilaterals.

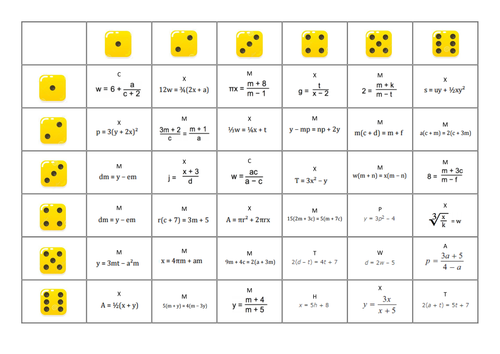
Rearranging Equations Revision Game
An opportunity to recap and revise simultaneous equations with a versatile resource.
Idea 1 -
Roll the dice to select the question and then race in pairs or small groups to gain the answer. Counters can be used to award point for the team with the first correct answer.
Idea 2 -
Connect 4. In pairs answer the question of your choice with the aim of forming 4 in a row.
Idea 3 -
In pairs take it in turns to roll a pair of dice and complete the question. A correct answer and you control the square, otherwise you partner has a turn to steal. Winner has the most squares.

Maths in Careers - National Careers Week
A selection of starters/do nows to aid National Careers Week and the application of mathematics to a wide variety of jobs.

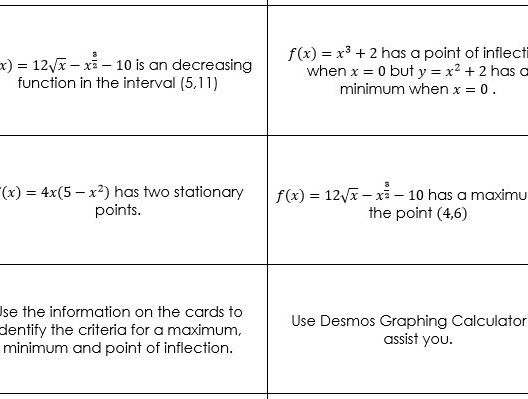
C2 Differentiation - Discovering Maximums, Minimums and Points of Inflection
Use the mix of 3 resources to introduce maximums, minimums and points of inflection. Students are encouraged to refer to graphs drawn via the free Desmos Graphing Calaculator and complete the set of rules they need to go on to tackle the chapter exercises or past exam questions. They'll also have a model answers to annotate and analyse for a method.

Product of Prime Factors - Relay Race
Prime factor decomposition is an deal opportunity to build it something a little different with an Optimus Prime factor decomposition relay. In pairs or teams students receive the first piece of the puzzle and are instructed to write the number as the product of its prime. On successful completion they receive the second piece and so on.
It culminate with a race to build the transformer that then leads to the ultimate conclusion that prime factorisation is simply about transforming numbers from one look to another without changing who they truly are!
PDF attached for ease and a word document attached so that you can edit the difficulty and create your own. The lesson that I typically accompany the activity with is also included.

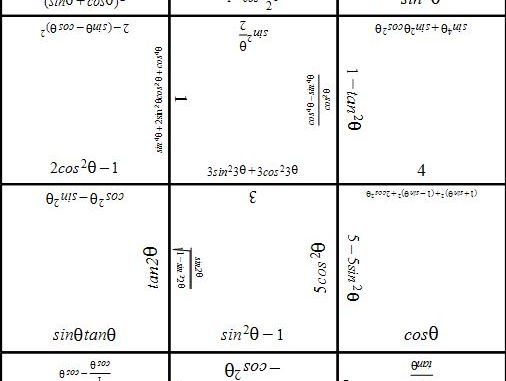
Trigonometric Identities Tarsia
A tarsia puzzle designed to develop students' use of trigonometric identities. Useful for both initial teaching and revision and recap for C2.
Involves numerous problems all requiring the application of the two most basic trigonometric identities.

Drawing Quadratics
Lesson crafted to enable all students to draw quadratics with the outcomes:
To substitute values into quadratics.
To draw quadratic graphs.
To find solutions to quadratics graphically.
Easily adaptable to more difficult quadratics.

Inequalities
Lesson designed to teach the following objectives in a high pace, clear progress and differentiated manner to a KS3 group.
To identify integer values of inequalities.
To show inequalities on a number line.
To read inequalities off a number line.
The first power point contains all worksheets, progress checks, extension tasks and quiz quiz trade cards. The second is edited just to include the slides that are presented to the students.

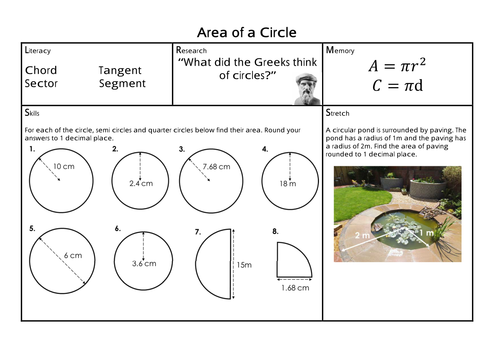
Area of a Circle - PRET Homework
An interpretation of Maths PRET homework (see http://prethomework.weebly.com/about.html for details) on the theme of area of a circle.

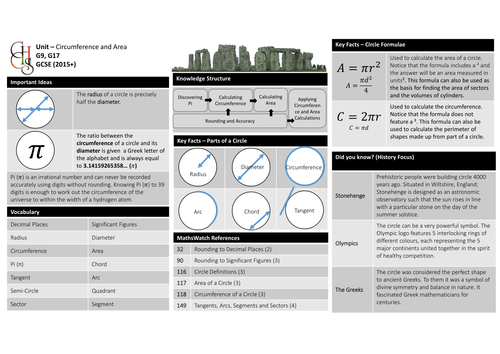
Knowledge Organiser - Area and Circumference
Another interpretation of 'Great Maths Ideas' work on knowledge organisers as a way of sharing learning intentions/objectives with students.
Please leave a comment.

Calculating the Interior Angles of a Polygon (Including Extension Problems)
A selection of problems around interior angles for students to solve. Follows on from the investigation into how side lengths link to angle sums. Leads into exterior angles and other problems.
EDIT: The first 4 slides for part of an Always, sometimes, never questioning session that I haven't obviously highlighted. I am fully aware that trapezium's don't have right angles. The task is designed to illicit misconceptions! Apologies for forgetting to signpost!

Probability - Expected Value
An opportunity to explore expected value through two way tables ( a potential examination combination). You'll find a modelling question, open task to explore, investigate and question as well as a extension question to really put the mathematics to good use. Enjoy!

Volume and Surface Area Relay
Relay for volume and surface area.
Students apply the formulae provided on the exam paper to the full range of 3D shapes. They also have an opportunity to consider surface area.

Circle Theorems
An extension of a NRich problem whereby students investigate a 10 dot circle (printout available from NRICH under printable resources).
The problem begins by consolidating isosceles triangles within a 10 dot circle. The problem then moves into exploring a series of circle theorems using the triangles they found from the first 2 problems.
Once students have done this they have the basis from which to generalise circle theorems.

Interior Angles
A selection of problems around interior angles for students to solve. Follows on from the investigation into how side lengths link to angle sums. Leads into exterior angles and other problems.

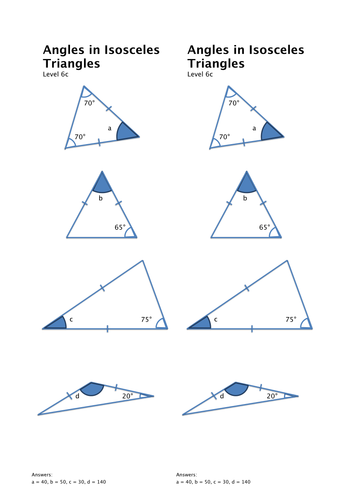
KS3 Angle Problems
From angles on a straight line through to isosceles triangles with a straight line.
Answers at the bottom. They can be chopped of or used to self assess with a focus on correctly justifying your answer as opposed to simply correctly answering.

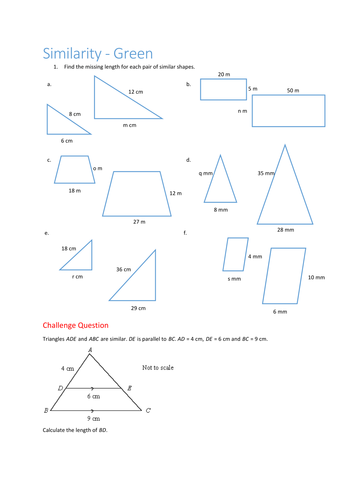
Similarity - Finding the missing length
Two differentiated worksheets on finding the missing lengths of similar shapes. Both worksheets conclude with a challenge question taken from GCSE exam papers.

Area of a Segment
I couldn't find a good resource on area of a segment so I spent some time developing one.
The idea is to provide students with 5 breadcrumbs that then lead to the final outcome, namely solving segment area problems.
The powerpoint has 5 steps that provide practice of all the assumed knowledge and this then enables student to guess the objective of the lesson and subsequently solve two A* questions taken from the &'Bland&'; question papers ( A* questions, Paper 2) which couldn't be linked.

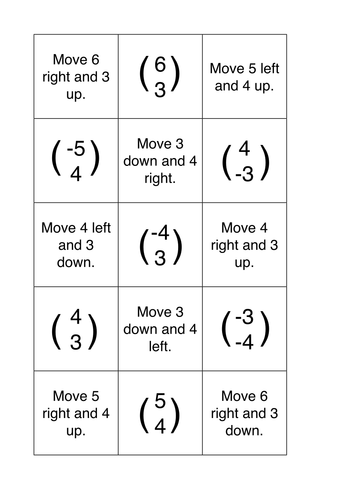
Transformations (with a focus on Translation)
A few resources I created a few years ago. They adapt the standard unit resources.