35Uploads
8k+Views
4k+Downloads
All resources

Elastic Collisions in Two Dimensions
This 19 slide PowerPoint presentation covers the content required for the
A-Level mathematics topic “Elastic Collisions in Two Dimensions”.
I developed it in order to teach the topic to my students.
The content is based on the Edexcel FM1 specification, and chapter 5 of the Edexcel FM1 textbook.
I have included extensive teacher notes with each slide.
I have extensively used PowerPoint’s animation effects. All slides use the “appear” animation to build up the content in a clear, meaningful manner.
I also used the “zoom”, “free motion” and “fade” animations to animate the collisions. This, I think, brings the material to life.
The slides contain all the required theory, and detailed, worked examples for each of the three sections:
Oblique Impacts with a Fixed Surface
Successive Oblique Impacts
Oblique Impacts of Smooth Spheres
Most examples are either fully original or extensively adapted/ modified from examples within the Edexcel textbook.
I have provided two free previews that you can download separately.
The first is an editable PowerPoint presentation containing an example of two spheres colliding, and concludes with an animation of the collision.
The second is a PowerPoint slideshow containing an example of a sphere colliding with a fixed surface. This example guides students through the use of the vector dot product.
I hope you find the PowerPoint very useful in your teaching practice.
Please feel free to contact me if you have any feedback.
Regards
Gerard

The Travelling Salesman Problem
This is a 50 slide PowerPoint presentation covering the content of Chapter 5 of Edexcel’s A-Level book “Decision Mathematics 1” (2017+ specification).
This includes:
Minimum Spanning Tree (Upper Bound)
Looking for Shortcuts
Residual Minimum Spanning Tree (Lower Bound)
Nearest Neighbour (Upper Bound)
[ I have also uploaded a free preview of this presentation to the TES website.]
The slide set makes extensive us of PowerPoint animations to clearly explain the involved algorithms.
I have provided teacher notes for most slides.
I have used an extended practical example throughout the presentation relating to a band’s (XTC) actual tour of the UK in 1979. The intent is to emphasis that the algorithm has practical uses in the real world.
Required terminology is revised, as is Kruskal’s and Prim’s algorithm’s for finding a Minimum Spanning Tree (MST).
I hope you find the PowerPoint presentation useful within your teaching.
Regards
Gerard

Hypothesis Testing and the Binomial Distribution
This 45 slide PowerPoint presentation takes you carefully through the contains of Chapter 7 of Edexcel’s Statistics and Mechanics Year 1/AS Book i.e. “Hypothesis Testing”.
I have uploaded a free preview of this presentation to TES so that you can see the level of detail, and presentation style, that I have used.
This chapter deals with performing Hypothesis Tests using the Binomial Distribution.
This presentation is ideal if you are using Edexcel’s recommend testbook
[ ISBN 978-1-292-23253-9 ].
However, the content is also very useful if you are using any other A Level textbook.
The presentation starts with a recap of the Binomial Distribution and how to compute probabilities from it. It shows how to directly compute probabilities from the Binomial Distribution, how to use the Cumulative Binomial Distribution tables, and how to use Casio calculators, both graphical (fx-9860, CG20 ,CG50) and scientific (fx-991EX).
It then covers one-tailed and two-tailed Hypothesis tests, both as a theoretical mathematical task, and within examination questions set within real-world contexts.
We cover both performing a Hypothesis Test directly, and calculating the Critical Values, Critical Regions and the Acceptance Region.
The Required Significance Level and the Actual Significance Level of the Hypothesis Test are clearly explained.
The two ways of performing a 2-tailed test Hypothesis Test are carefully explained i.e. strictly requiring that each critical region has a probability less than alpha/2, and making each critical region have a probability that is as close as possible to alpha/2.
Teacher notes are provided for each slide.
I have also included my solutions to all the exercises in the chapter. I have not tried to do the questions in the same way as the solution bank, so I think you will find my solutions useful.
Errors in the chapter, the book’s answers and in the solution bank are pointed out.
The presentation extensively uses “Appear on Click” so that you can step the students through the contents of each slide. No other animations are used as I think these can become an unnecessary distraction.
I hope you find the resource very useful within your teaching practice.
I am very happy to receive any feedback you might have.
Regards and best wishes
Gerard

Linear Programming for GCSE / Core Maths
I have always found it difficult to get across the concepts required to support Linear Programming to my weaker Higher GCSE groups and especially, more recently, my Core Mathematics groups.
There are several issues preventing the less confident mathematicians from doing well in this topic. Firstly, many students are not even confident about plotting simply straight lines such as x = 3, y = 7, x = y etc. Secondly, a lot of them have not fully understood the concept of an inequality. Thirdly, in general these students find it very difficult to convert an English sentences into mathematical statement.
I have put together this 90+ PowerPoint slide set to address these problems.
I have created interesting/funny ways to remember that x = 3 is a vertical line, whilst y = 3 is horizontal. I have included practice on plotting straight lines and shading regions represented by inequalities. I also introduce the idea of a “test point” that will allow stidents to work out which side of the line they require.
I have included practice on solving simultaneous equations using a
Casio fx-991EX CLASSWIZ calcuator, and also by manulally solving the equations. A video of how to use the Casio 991 to solve simultaneous equations in included.
Of course, the idea of an Objective Function covered, together with how to use the feasibility region to locate the required minimum/maximum value.
I focus the students on investigating points close to or at the vertices of the feasibility region. I have stayed away from moving an example objective line through the feasibility region to see which is the last vertex it goes through. This proved a bridge too far for too many of my students!
The slide set includes a lot of worked examples, and practice questions along with their solutions.
I have also uploaded a free preview PowerPoint Slideshow that contains approximately half of the slides.
I hope you find the presentation useful. Please let me know if you find any typos or if you have a suggestion as to how it can be improved.
Regards
Gerard

Floyd's Algorithm
When I had to teacher Floyd’s algorithm for the first time, I found that the available resources made it seem more difficult than it really was. This is because of unclear descriptions within the textbook about how to work simultaneously with both a distance and route tables.
I therefore decided to create this fully animated PowerPoint presentation that clearly shows how the two tables are simultaneousily managed as you work towards the final solution.
The 31 slides are the result of many hours of using PowerPoint’s more advanced features to provide a detailed explanation of the algorithm by working through every step of a typical example question.
(Students will not realise that so many slides are being used “behind the scene”.)
I have also provided extensive teacher notes throughout the slides.
I have made full use of PowerPower’s animation features to bring the algorithm to life. Each decision about updating the distance and route tables is clearly shown, and the updates are done using “fade in”, “fade out” and “motion path” animations.
Once the final distance and route tables are achieved, I dynamically build the optimised routes from the information in the route table.
The presentation could be given to students as a self-paced learning task, or used as the centrepiece of a teacher led lesson.
I have provided a free preview PowerPoint Slideshow so that you can see how clear Floyd’s Algorithm becomes once you make each decision more dynamic, explicitly showing how the distance and route tables are simultaneously updated.
I hope you find this resource very useful. I am happy to act upon any feedback you have as to how I could further improve it.
Regards
Gerard

The Roots of Polynomials
This PowerPoint presentation (of 24 slides) covers the relationship between the roots of quadratic, cubic and quartic polynomials and their coefficients.
These relationships are derived algebraically, and worked examples are provided explaining typical A-level questions.
If you want to try out this presentation, you can download a free, sample PowerPoint slideshow.
Slides are included to remind students of how the graph of the polynomial is related to the number of real roots, including repeated roots.
The standard results for the “sum of reciprocals”, “sums of squares” and “sum of cubes” of the roots of quadratics, cubics and quartics are derived and used.
The presentation finishes with applying the newly learned knowledge to graph transformations.
I have kept the presentation style as simple as possible, using the “appear” animation extensively to build up each slide.
I have also included teacher’s notes for each slide.
In summary, this PowerPoint presentation provides everything you need to teacher this A-level Further Mathematics topic.
I hope you find this resource useful within your teaching practice.
I would be very happy to receive any feedback you might have.
Regards and best wishes,
Gerard

Methods in Calculus PowerPoint Presentation- Edexcel, Core 2, Chapter 3
This PowerPoint Presentation covers the contents of chapter 3 - “Methods in Calculus” - from Edexcel, Core 2.
Topics include:
Improper Integrals
The Mean Value of a Function
The Mean Value and Graph Transformations
Differentiation Inverse Functions (e.g. arcsin), and Associated Integrals
Integration and Partial Fractions.
You can adapt the contents to your own teaching.
If you just want to use the slides without making any changes, you can download the associated PowerPoint Slideshow from TES free.
Regards and best wishes,
Gerard

The Central Limit Theorem - PowerPoint Presentation - Edexcel, Further Statistics 1, Chapter 5
This PowerPoint Presentation covers The Central Limit Theorem for Edexcel, A-Level Further Statistics 1, Chapter 5
The contents cover:
The Central Limited Theorem in overview
The general application of the Central Limit Theorem
Applying the Central Limit Theorem to other distributions
e.g. the Poisson Distribution and the Negative Binomial Distribution
The presentation is fully editable for use as a teaching / learning aid in your own practice.
If you do not want to adapt its contents, the associated PowerPoint Slideshow is available to download for free from TES.
Regards and best wishes
Gerard

The Poisson Distribution - PowerPoint Presentation - Edexcel, Further Statistics 1, Chapter 2
The PowerPoint Presentation covers the contents of Chapter 4 of Edexcel’s Further Statistics 1 textbook.
The contents cover:
How the Poisson Distribution can be derived from the Binomial Distribution
Formal Definition of the Poisson Distribution
Alternative Derivation of the Poisson Distribution
Modelling with the Poisson Distribution
Adding Poisson Distributions
The Mean and Variance of a Poisson Distribution
Using the Poisson Distribution to model a Binomial Distribution
This presentation can be adapted / amended to use as a teaching / learning aid. If you do not want to adapt its contents, the associated PowerPoint Slideshow is available to download for free from TES.
Regards and best wishes
Gerard

Mathematical Logic for TMUA Paper 2 - PowerPoint Presentation (68 slides)
This 68 slide PowerPoint Presentation covers all the Mathematical Logic content required for TMUA Paper 2.
It closely follows the content within the PDF resource provided by UAT UK.
However, the PowerPoint slides build up in a way that makes the topic more easily understood (in my humble opinion).
If you do not need to adapt the contents, you can download the associated PowerPoint Slideshow for free from TES.
Regards and best wishes,
Gerard

The Exponential Distribution - PowerPoint Presentation - AQA, OCR, ...
This PowerPoint Presentation covers the Exponential Distribution with respect to A-Level Further Statistics.
The topics coveed are;
The connection between the Poisson and Exponential Distributions
The formal definition of the Exponential Distribution
The Probability Density Function (PDF) of the Exponential Distribution
The Memoryless Property of the Exponential Distribution
Finding probabilities using the PDF
The median of the Exponential Distribution
The IQR of the Exponential Distribution
The Expected Value, E(X), of the Exponential Distribution
The Standard Deviation of the Exponential Distribution
You can fully adapt the presentation to your own teaching / learning requirements once you have downloaded it for £2.
If you do not want to change its contents, the associated PowerPoint Slideshow is available to download for free from TES.
Regards and best wishes
Gerard

Hypothesis Testing: Poisson / Geometric Distributions - Edexcel, Further Statistics 1, Chapter 4
This PowerPoint Presentation covers the contents of chapter 4 (Hypothesis Testing) from Edexcel, Further Statistics 1.
Topics covered:
Hypothesis Testing and the Poisson Distribution
Hypothesis Testing and the Geometric Distribution
Critical Regions and Critical Values
If you do not want to adapt the contents, you can download the associated PowerPoint Slideshow for free from TES.
Regards and best wishes,
Gerard

Geometric and Negative Binomial Distributions - Edexcel, Further Statistic 1, Chapter 3
This PowerPoint Presentation covers the contents of chapter 3 - " Geometric and Negative Binomial Distributions"- from Edexcel, Further Statistics 1.
Topics:
The Geometric Distribution
The Mean and Variance of a Geometric Distribution
The Negative Binomial Distribution
The Mean and Variance of a Negative Binomial Distribution
If you do not want to adapt the contents, you can download the associated PowerPoint Slideshow for free from TES.
Regards and best wishes,
Gerard

Differentiation A-Level Year 2 - Full 93 Slide Presentation
This is a 93 slide PowerPoint presentation that covers all the content of A-Level Year 2 Differentiation.
Full notes are provided for each slide, and a free preview presentation, containing approximately half the slides, is also available.
We start with an introduction to connected rates of change using examples related to a sponsored charity walk. The examples provide students with an understanding that if one rate of changes leads on to a second rate of change then we obtain the overall rate of change by multiplying the two connected rates of change.
However, the foundation of Year 2 Differentiation is the Chain Rule, and how to use it to differentiate composite functions. Students struggle with the Chain Rule if they do not first have a solid grasp of composite functions. With this in mind, we remind students of how composite functions are created out of simpler functions.
We then provide an example of differentiating a function (a composite function) using our Year 1 method i.e. expanding brackets before differentiating. This example provides the opportunity to ask whether there is a quicker/simpler way of doing such a differentiation.
The term one-stage composite function is used for a composite function that can be differentiated without first having to simplify the expression. This leads to a two-stage composite function, and a proof of The Chain Rule.
Plenty of examples, and practice questions, are provided for the Chain Rule.
We then cover using the Chain Rule to prove that the rate of change of x on y is the reciprocal of the rate of change of y on x.
We next prove the Product and Quotient Rules, and provide various examples of combining them with the Chain Rule.
Next we expand the range of functions that we can differentiate from first principles, starting with trigonometric functions (small angle approximations are also covered).
Exponential functions are next, leading to the fundamental constant e, and the realisation that the gradient of the graph y=e^x at any point (x, y) equals the y-coordinate at that point.
A spreadsheet is provided so that trial and improvement can be used to guide students to the value of e.
We next derive the differential of a^x and ln x.
This is a great opportunity to highlight this result as the missing piece from Year 1 differentiation i.e. we have now found the curve whose gradient is 1/x.
The next two sections provide further applications of the Chain Rule i.e. parametric equations and implicit differentiation.
The definitions of Concave, Convex and Point of Inflection are covered. The slide set goes further than the textbook, discussing the use of the 3rd differential.
The final section returns to where we began, namely Connected Rates of Change.
I hope you find the slide set useful in your teaching. I would be very pleased to receive any comments, or requests for improvements.
Regards
Gerard

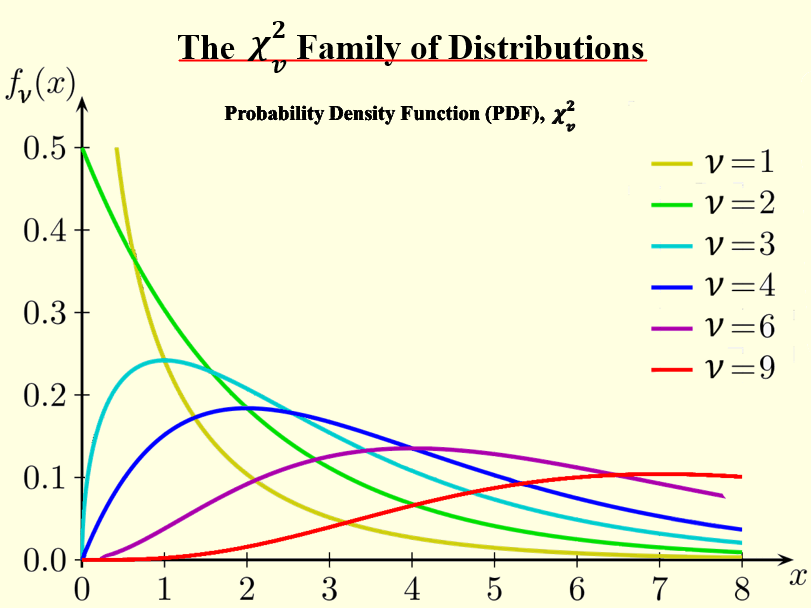
Chi-Squared Tests (aka Goodness of Fit)
Chi-Squared Tests for Goodness of Fit
This 58 slide PowerPoint presentation covers the content of “Chi-squared Tests” within Edexcel Further Statistics 1 (Chapter 6 of the official Pearson FS1 textbook).
Each slide is accompanied by teacher notes.
[A free preview PowerPoint slideshow, containing approximately half the slides, is also available.]
We start with a general introduction for how you quantity the closeness of observed frequencies to the frequencies expected for a chosen standard distribution i.e. how to calculate Pearson’s Cumulative Test Statistic, X^2.
Both methods of calculating X^2 are covered.
In order to properly understand the concept of “Degrees of Freedom”, additional examples are included to fully explain how we determine the degrees of freedom of a given dataset by taking into account restrictions placed upon it. In my opinion, this is handled poorly by the textbook.
The loss of degrees of freedom due to having to combine adjacent columns of the dataset (because the observed/expected frequencies are too low), and because the observed data itself is needed to calculated a required parameter of the model distribution is also covered.
We then link this to our existing knowledge of Hypothesis Tests, and introduce the Chi-squared family of distribution. This section has also been extended to ensure that students properly understand how the chi-squared graphs vary with the data’s degrees of freedom, ν, and how to effectively these graphs (either by using the table “Percentage Points of the Chi-Squared Distribution” in the formula book or by using a calculator e.g. the Casio CG50).
Once we have the foundations in place, we use them to work through exam-style examples, comparing the given observed data to a chosen standard model distribution. For each example, we perform a hypothesis test to determine if there is sufficient evidence, at the required significance level, to reject null hypothesis.
The examples cover the following standard distributions:
Discrete Uniform Distribution
Geometric Distribution
Binomial Distribution
Poisson Distribution
_
All examples are either newly created or significantly modified from past paper questions.
For each standard distribution we explain how to determine the probability of each outcome and, hence, the expected frequencies. We also explain how to, if necessary, estimate a required parameter (e.g. ρ for the Binomial distribution, λ for the Poisson Distribution) from the observed data.
The presentation, unlike the textbook, deals with the four standard, discrete distributions in turn i.e. it does not delay the section on the Geometric Distribution until the end. Instead, the section on continency tables is left to the end and given its proper title of “The Chi-Squared Test of Independence”.
In addition, the presentation contains detailed examples showing how to use the Casio CG50 calculator to perform Chi-Squared tests.
Regards
Gerard

Linear Programming: The Two Stage Simplex and the Big-M Algorithms
These two PowerPoint presentations fully explain the ideas behind the
Two-Stage Simplex and the Big-M Algorithms.
They both provide clear, animated, step-by-step examples explaining how to apply the algorithms.
[ I have also separately uploaded a free preview so that you can see the style of the presentation. ]
Slack, surplus and artificial variables are explained, as is the creation of the additional objective function (i.e. A = “The total of the artificial variables”), and the modification of the original objective function when using the Big-M method.
The slides can be used as the basis for teaching the topic to an A-Level mathematics class, and they also clear enough to be used as a revision/self-study aid.
For example, "triggers " are used to show how values within a tableau are calculated using row operations on the previous tableau.
Extensive use has been made of PowerPoint’s animation features such as “appear”, “fade” etc…
I hope this resource will be useful within your teaching, and I would happy to receive any feedback that you might have.
Regards and best wishes
Gerard

Probability Generating Functions
This 34 slide PowerPoint presentation covers the contents of Edexcel FS1 Chapter 7 “Probability Generating Functions”.
(I have also uploaded a free, 16 slide, preview presentation that shows you the level of detail contained within the slides, and the presentation style.)
The presentation includes many new examples and proofs of the results covered within the topic.
The full slide set also comes with my solutions to all the questions of every exercise (including the “Challenges”) within the chapter.
(I do the questions independently from any published solutions, so you might find I have arrived at the same answer in a different way, showing detailed working along the way.)
I am happy to receive any feedback you might have for further improvements to the presentation.
Regards
Gerard

Differentiation A-Level Year 2 (Free Sample)
This is a free sample of my 93 slide PowerPoint presentation that covers all the content of A-Level Year 2 Differentiation. The notes pages for the full presentation are also provided.
We start with an introduction to connected rates of change using examples related to a sponsored charity walk. The examples provide students with an understanding that if one rate of changes leads on to a second rate of change then we obtain the overall rate of change by multiplying the two connected rates of change.
However, the foundation of Year 2 Differentiation is the Chain Rule, and how to use it to differentiate composite functions. Students struggle with the Chain Rule if they do not first have a solid grasp of composite functions. With this in mind, we remind students of how composite functions are created out of simpler functions.
We then provide an example of differentiating a function (a composite function) using our Year 1 method i.e. expanding brackets before differentiating. This example provides the opportunity to ask whether there is a quicker/simpler way of doing such a differentiation.
The term one-stage composite function is used for a composite function that can be differentiated without first having to simplify the expression. This leads to a two-stage composite function, and a proof of The Chain Rule.
Plenty of examples, and practice questions, are provided for the Chain Rule.
We then cover using the Chain Rule to prove that the rate of change of x on y is the reciprocal of the rate of change of y on x.
We next prove the Product and Quotient Rules, and provide various examples of combining them with the Chain Rule.
Next we expand the range of functions that we can differentiate from first principles, starting with trigonometric functions (small angle approximations are also covered).
Exponential functions are next, leading to the fundamental constant e, and the realisation that the gradient of the graph y=e^x at any point (x, y) equals the y-coordinate at that point.
A spreadsheet is provided so that trial and improvement can be used to guide students to the value of e.
We next derive the differential of a^x and ln x.
This is a great opportunity to highlight this result as the missing piece from Year 1 differentiation i.e. we have now found the curve whose gradient is 1/x.
The next two sections provide further applications of the Chain Rule i.e. parametric equations and implicit differentiation.
The definitions of Concave, Convex and Point of Inflection are covered. The slide set goes further than the textbook, discussing the use of the 3rd differential.
The final section returns to where we began, namely Connected Rates of Change.
I hope you find the slide set useful in your teaching. I would be very pleased to receive any comments, or requests for improvements.
Regards
Gerard

Floyd's Algorithm (Free Preview)
This is a free, 11 slide, preview, of my full PowerPoint slide-set on Floyd’s Algorithm (also on TES, cost = £2).
When I had to teacher Floyd’s algorithm for the first time, I found that the available resources made it seem more difficult than it really was. This is because of unclear descriptions within the textbook about how to work simultaneously with both a distance and route tables.
I therefore decided to create this fully animated PowerPoint presentation that clearly shows how the two tables are simultaneousily managed as you work towards the final solution.
The 31 slides are the result of many hours of using PowerPoint’s more advanced features to provide a detailed explanation of the algorithm by working through every step of a typical example question.
(Students will not realise that so many slides are being used “behind the scene”.)
I have also provided extensive teacher notes throughout the slides.
I have made full use of PowerPower’s animation features to bring the algorithm to life. Each decision about updating the distance and route tables is clearly shown, and the updates are done using “fade in”, “fade out” and “motion path” animations.
Once the final distance and route tables are achieved, I dynamically build the optimised routes from the information in the route table.
The presentation could be given to students as a self-paced learning task, or used as the centrepiece of a teacher led lesson.
I have provided a free preview PowerPoint Slideshow so that you can see how clear Floyd’s Algorithm becomes once you make each decision more dynamic, explicitly showing how the distance and route tables are simultaneously updated.
I hope you find this resource very useful. I am happy to act upon any feedback you have as to how I could further improve it.
Regards
Gerard

Elastic Collisions in Two Dimensions - Preview 2
This is a free preview of my full Powerpoint presentation for “Elastic Collisions in Two Dimensions”.
It contains an example of an oblique collision between a sphere an a fixed surface. It also guides students through the use of the vector dot product.
The file is in PowerPoint slideshow format so that you can see the animation of the collision and the build up of the solution.
The full 19 slide PowerPoint presentation covers the content required for the
A-Level mathematics topic “Elastic Collisions in Two Dimensions”.
I developed it in order to teach the topic to my own students.
The content is based on the Edexcel FM1 specification, and chapter 5 of the Edexcel FM1 textbook.
I have used PowerPoint’s animation effects extensively.
All slides use the “appear” animation to build up the content in a clear, meaningful manner. I also used the “zoom”, “free motion” and “fade” animations to bring the collisions to life.
I have included extensive teacher notes with each slide.
The slides contain all the required theory, and detailed, worked examples for each of the three sections:
Oblique Impacts with a Fixed Surface
Successive Oblique Impacts
Oblique Impacts of Smooth Spheres
Most examples are either fully original or extensively adapted/ modified from examples within the Edexcel textbook.
I hope you find the material useful in your teaching of the topic.
Please feel free to contact me if you spot any typos.
Regards
Gerard